- 4.6 Connecting Graphs Of F F' F'ap Calculus 2nd Edition
- 4.6 Connecting Graphs Of F F' F'ap Calculus Calculator
Answer
4.6 Connecting Graphs Of F F' F'ap Calculus 2nd Edition
$limlimits_{h to 0}frac{f(x+h)-f(x-h)}{2h} = f'(x)$ The diagram shows that the slope of the line connecting the points $(x-h, f(x-h))$ and $(x+h, f(x+h))$ approaches the slope of the graph at $x$ as $hto 0$


F is differentiable at x = 2. The derivative of f is continuous at x =2. (A) I only (B) II only (C) I and II only (D) I and III only (E) II and III only 80. Let f be the function given by f 2xe= 4x2. For what value of x is the slope of the line tangent to the graph of f at x,fx equal to 3? 62 Connecting f’ and f” with the graph of f. First derivative test for local extrema 4.3a: 3 – 6, 37, 40, 43, 45 63 Connecting f’ and f” with the graph of f. Concavity. Second derivative test for local extrema 4.3b: 1, 29, 33, 34, 41, 42, 44 64 Connecting f’ and f” with the graph of f. Hack fx factory mac os. Free gameswhy does microsoft hate me?. Calculus Grapher (A nifty tool to see the relationship between functions and their derivatives).
4.6 Connecting Graphs Of F F' F'ap Calculus Calculator
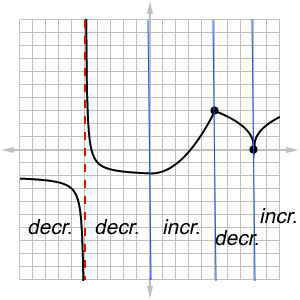
Work Step by Step
$limlimits_{h to 0}frac{f(x+h)-f(x-h)}{2h} = frac{0}{0}$ We can apply L'Hospital's Rule. $limlimits_{h to 0}frac{f'(x+h)-(-1)f'(x-h)}{2} = frac{2f'(x)}{2} = f'(x)$ The diagram shows that the slope of the line connecting the points $(x-h, f(x-h))$ and $(x+h, f(x+h))$ approaches the slope of the graph at $x$ as $hto 0$
